Working Papers explore the technical and scientific themes that underpin GNSS programs and applications. This regular column is coordinated by Prof. Dr.-Ing. Günter Hein, head of Europe’s Galileo Operations and Evolution.
Working Papers explore the technical and scientific themes that underpin GNSS programs and applications. This regular column is coordinated by Prof. Dr.-Ing. Günter Hein, head of Europe’s Galileo Operations and Evolution.
Can precise positioning only be performed by high-end dual-frequency GNSS receivers? Many in the GNSS community will answer in the affirmative because of a prevailing impression that accurate results essentially require dual-frequency receivers — preferably using carrier phase measurements for precise positioning applications. This way of thinking is primarily motivated by the fact that the (first order) ionospheric delay can be eliminated by the use of at least two frequencies and carrier phase measurements are less affected by multipath effects than range measurements.
In the past many single-frequency approaches have been proposed to obtain precise results from a low-cost single-frequency GNSS receiver. However, the main obstacle to achieve precise single-frequency positioning with the current core GNSS signals (GPS and GLONASS) remains the high level of code range noise, substantially poisoned by multipath errors, which can be a few decimeters up to some meters. Further, the high level of multipath effects on the currently available frequencies (e.g. GPS and GLONASS L1 and L2 bands) makes precise single-frequency positioning impossible.
Performing single-frequency positioning with Galileo E5 could offer a possibility to realize precise positioning solutions with moderate budget. Note that positioning is not limited to land applications only. A number of satellite missions require fairly accurate orbits and GPS single-frequency receivers have become an important means of orbit determination, whereas only a few dual-frequency GPS receivers are in orbit (mainly limited to certain types of could offer an interesting future field for Galileo E5 single-frequency receivers.
Galileo E5: Broadband AltBOC Signal
A major innovation in signal technology is about to come from Europe’s Galileo system, which provides a unique broadband signal with a nominal bandwidth of more than 90 megahertz and a center frequency of 1191.795 MHz. (Note, however, that the authorized bandwidth is 51.15 megahertz according to the Galileo Signal in Space Interface Control Document.)This signal (but not its sub-carriers E5a and E5b) features a very low noise figure at centimeter level.
The time series illustrated in Figure 1 (at the top of this article) was computed by a subtractive combination of code range and carrier phase measurements to obtain the code-range noise (including ionospheric variations) and portrays the values of Galileo E1 and E5 collected from the GIOVE-B experimental Galileo satellite. As the figure data show, the level of code-range noise for Galileo E5 is extraordinarily low — much lower than that for Galileo E1. The multipath errors for Galileo E5 are reduced by a factor of three to five (see Table 1).
These characteristics open the possibility of performing code-range measurements at the centimeter level and enable a better mitigation of multipath effects. The drastically increased range precision due to the very low E5 range noise allows for obtaining more accurate combined code-and-carrier observables. The minimum we are expecting from a modern high-quality receiver is the use of “narrow correlator” or equivalent technologies to mitigate multipath effects. The corresponding models to the use of “narrow correlator” technology are the basis to generate simulated (“synthetic”) data for the positioning experiments using a GPS/Galileo software receiver.
Galileo E5 AltBOC Receiver
The implementation of a Galileo E5 capable single-frequency receiver must be carried out under the consideration of all major requirements for precise positioning, in particular with respect to bias-free measurements, noise mitigation, and internal multipath mitigation in the receiver. In this study we followed two technology approaches to implement such a receiver, namely an FPGA-based and a software (s/w)-defined receiver. While the first approach acquires the E5 signal through a wide-band front end and thus allows direct demodulation, the second one uses a dual-front end to receive the lower E5a and the upper E5b band separately.
To achieve the requested flexibility and configurability, a software receiver appears to be the right technical solution. To keep costs low, the software part has to be linked to a suited RF-front end hardware receiver part, to support single-frequency Galileo E5 operations. Figure 2 shows a block diagram of the s/w receiver implementation scheme.
Code-Plus-Carrier (CPC)
An additive combination of pseudorange and carrier phase measurements (the code-plus-carrier: CPC principle) can completely eliminate the ionospheric delay (a major point of uncertainty in precise positioning) due to the fact that group and phase delay have opposite signs) as illustrated in Figure 3 and reflected in the following equation:
Equation 1 (see inset photo, above right)
where ρ is the pseudorange measurement, ϕ is the carrier phase measurement in metric units, r is the geometric distance between satellite and receiver antenna, λ is the wavelength of the Galileo E5 carrier, N is the ambiguity parameter, δT is the tropospheric delay, δMCPC is the influence of multipath on the measurement, and εCPC are the additional code and carrier phase noises.
This method was basically recognized but rarely applied to terrestrial position determination. Indeed, the method was proposed in 1996 under the name of GRAPHIC (Group and Phase Ionosphere Correction) for GPS orbit determination because the majority of space-capable receivers are of single-frequency type. In contrast to traditional pseudorange positioning, here we must deal with the unknown position and also the unknown ambiguity parameter (as in carrier phase positioning) in the newly built observable. This requires a longer observation window in order to allow sufficient convergence of the ambiguity parameters.
In practice, we make use of double differences in order to minimize the satellite and receiver clock errors and to attenuate other sources of errors. For this purpose, access to a global or continental network (e.g., International GNSS Service or EUREF) is sufficient as experience has shown that the use of regional networks (shorter baselines) will not further improve the positioning accuracy.
Tropospheric delays can still compromise the positioning accuracy. For this reason, either external sources providing precise corrections (e.g., numerical weather models) should be incorporated or make use of additional tropospheric delay parameters in the estimation process. Multipath errors are site-specific and particularly strong on the code ranges. Here, the use of E5 AltBOC provides a key advantage, as this broadband signal shows an ultra-low multipath behavior compared to all other GNSS signals.
Performance Assessment of the Single-Frequency Positioning with E5
The fundamental work here is to show the benefit of Galileo E5 regarding single-frequency positioning compared to single-frequency positioning using GPS L1 or L5 as well as to traditional multi-frequency carrier phase processing. Figure 4 illustrates the processing procedures of the Galileo E5 single-frequency data. All experiments are based on simulated GNSS data because the number of existing Galileo satellites broadcasting the E5 signal at the moment is not sufficient to provide real-world data. The simulated data (Galileo as well as GPS data) have been generated using an own implemented application (Nereus).
The results are analyzed by a set of different statistical methods to determine the achievable accuracies of the single-frequency positioning approach using Galileo E5. In generating the results we assumed a full Walker 27/3/1 Galileo constellation, which will be available by 2020 according to the current state of development.
CPC Results for Galileo E5 versus GPS L1
We carried out an analysis of two daily (24 hour) data batches of Galileo E5 and GPS L1 signals using a combination of code and carrier measurements with a sampling rate of 30 seconds to assess the expected positioning performance. A pre-analysis using real and synthetic GPS L1 data showed a scaling factor of 1.06 between the synthetic and the real datasets. Hence, the synthetic data reflect as much as possible the reality.
The Galileo E5 coordinate components yielded results in the range of a few centimeters. The horizontal components were around 2 centimeters and better than the vertical components, which ranged from 3 to 5 centimeters. One of the reasons for this is the higher dilution of precision of the vertical component (VDOP).
The daily data batches for Galileo E5 single-frequency yielded a total error of around 3–5 centimeters 3D RMS depending on the measurement environment whilst for GPS L1 they yielded up to 20 centimeters 3D RMS. The great advantage of using Galileo E5 signals to perform CPC single-frequency positioning can readily be seen at a glance in Figure 5. This figure represents the time series of Galileo E5 and GPS L1 and the amplitude of the single shots of Galileo E5 is at the low centimeter level.
Another experiment involved showing the ability of Galileo E5 (AltBOC) CPC results to detect position change in a moving structure over an extended period of time. The average motion rate for a structure such as a rock glacier is expected to range around 70 centimeters per year, see Figure 6. The reference period is set to day 206 in the year 2011, and data were continuously generated.
After 64 days the displacement rate between days 206 and 270 was calculated. The data were computed for GPS L1 and Galileo E5 using the single-frequency CPC principle with the results given as point scatter plots in Figure 7. These plots draw a clear picture of the relative detection ability of the two datasets. By analyzing the statistical distribution of the measurements, we see that the GPS L1 measurements have an error ellipse of around 30 centimeters, which is not sufficient to detect any significant change in the position of the station if we consider an expected motion rate of about 11 centimeters for that observation period as given by Figure 6. The single shots of the coordinates for the two periods overlap (blue and green dots).
For Galileo E5 measurements, a clear difference between the two periods of observation is perceptible, which indicates that the station has undergone a displacement. The positioning accuracy is still too low to depict a clear behavior of the movement because of a five-centimeter error ellipse of the Galileo E5 results. Nonetheless, the Galileo E5 single-frequency results are accurate enough to detect a position change after a relatively short period of time.
After 126 days of data logging, we repeated the experiment for the same station. The point scatter plots on Figure 8 depict the results. Now the displacement of the station is much more noticeable with Galileo E5 measurements.
Using Galileo E5 CPC single-frequency positioning, we are able to detect deformation or displacement in the range of a few centimeters to decimeters. CPC positioning using a Galileo E5 single-frequency receiver has a certain advantage here compared to carrier-phase processing. Because of the moderate price of a single-frequency system, we can use more sensors to determine an exact profile of the deformation. Due to the long convergence time (20–30 minutes), single-frequency positioning using the CPC principle could be suited for certain types of landslide or glacier monitoring applications, because changes in such structures can only be detected after a sustained period of observation.
CPC Results for Galileo E5 versus GPS L5
GPS modernization provides a new civil signal at the L5 frequency. This signal is intended to increase precision and robustness of the navigation solution as the result of mitigation of ionospheric refraction errors and implementation of an enhanced design with higher signal strength and advanced code structure compared to the existing GPS civil signals.
The L5 signal is improved compared to GPS L1 and has the same center-frequency (1176.45 MHz with a 24 MHz bandwidth) as the E5a sub-carrier of the Galileo broadband E5 signal. Hence, L5 seems to have a similar characteristic to at least one part of the E5 signal.
Using the same procedures as before, we generated and processed GPS L5 and Galileo E5 synthetic data. Comparing the results confirmed that GPS L5 is more robust than L1 regarding the effect of code noise. The RMS values of the CPC results using GPS L1, L5, and Galileo data are shown in Figure 9. The graph presents results for 1-, 3-, 6-, and 24-hour data batches.
As with the earlier experiments, the GPS L1 positioning results are outside of the range that we could call precise. Even with a daily data batch, one can only reach a decimeter to sub-decimeter level of positioning accuracy. Using GPS L5 produces a noticeable improvement, but the results are still at least two times worse than those for Galileo E5.
The results show the need for a certain convergence time to obtain precise coordinates due to the presence of ambiguity terms in the CPC observables. For example, one might have to gather measurements for at least one hour to achieve sub-decimeter precision for Galileo E5. This relatively long convergence time must be properly addressed by fixing the ambiguity terms to their integer values.
These tests assessed the performances of the Galileo E5 CPC algorithm and showed a 3D positioning accuracy of around 5 centimeters in critical environments and 2–3 centimeters under normal conditions by processing daily data batches. In comparison, these results are four to five times better than GPS L1 and two to three times better than GPS L5.
Regarding the first obtained results, the single-frequency positioning concept using the potential of Galileo E5 clearly shows some innovative aspects with a definite potential to be developed. Due to its very low code range noise and the even lower multipath influence on the positioning solution (compared to other GNSS signals like GPS L1 or L5), the Galileo E5 CPC single-frequency results are able to fulfill the requirement for many GNSS precise positioning applications.
E5 CPC versus Carrier Phase Processing
Many precise GNSS positioning applications rely on carrier phase tracking because of the very low measurement noise and low multipath effects. As a result, carrier phase processing accuracy reaches the millimeter level.
A user equipped with a multi-frequency GNSS receiver can estimate the ionospheric group delay and phase advance from the measurements, and essentially eliminate the ionosphere as a source of measurement error. A relative ionosphere-free linear combination of carrier phase measurements can properly eliminate the ionospheric error and delivers results at the millimeter level. Using such a combination for different GPS signals (L1+L2, L1+L5) the results are compared with the CPC Galileo E5 results. As we see in Figure 10, the results obtained with the carrier phase combination are much more accurate than the ones with Galileo E5 CPC. The difference between the two results is scaling around a factor of 10.
Even though Galileo E5 CPC results cannot be compared to multiple frequency results (Figure 10) in terms of accuracy, the CPC approach can still meet the requirements of various precise positioning applications requiring decimeter- and centimeter-level accuracies. Moreover, not every precise positioning application requires millimeter-level accuracy with the associated high cost expenditure for a multi-frequency receiver. Hence, the CPC approach can fill a niche between highly precise positioning using multi-frequency carrier phase processing and conventional single-frequency positioning.
CPC Ambiguity Resolution
The basic principle of the CPC algorithm uses two completely different types of measurements to build a new observable: the code range measurements, which are much noisier and affected much more severely by multipath, and the carrier phase measurements, which contain ambiguity terms. This ambiguity term needs to be fixed to an integer value in order to produce precise results.
Until now the results only used estimated float solutions of the ambiguity terms. This estimation does not solve the problem, because the results are not sufficient to obtain precise positions quickly. Furthermore, use of float solutions means that a certain observation time is needed to converge to reasonable values. This assumes results at the decimeter level after around 30 minutes and centimeter level after 3 hours.
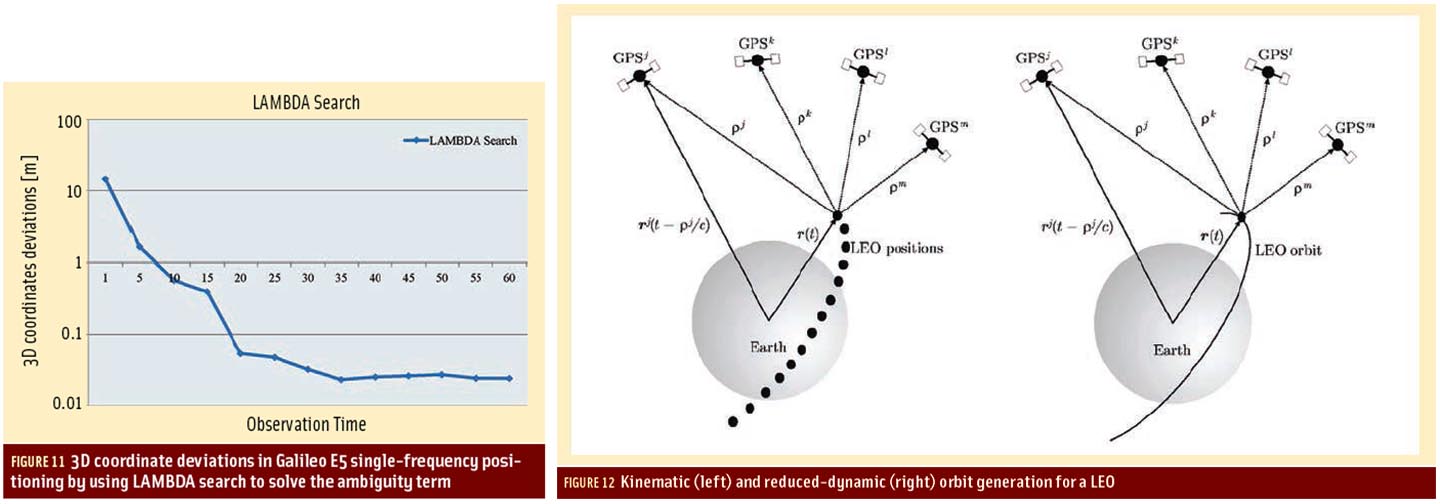
So, resolving the unknown ambiguities of the double differenced CPC observable is the key to rapid and very precise GNSS positioning. Therefore it is imperative to fix the ambiguity term to its integer value. Many algorithms can resolve the ambiguity term. The “Least-squares AMBiguity Decorrelation Adjustment” (LAMBDA) is a very efficient method used to fix the ambiguity and is less intensive in the computation than other methods.
Due to the high level of noise in the GPS L1 code measurements, it is difficult to resolve the ambiguity term in the newly built CPC observable for this signal. Using the LAMBDA algorithms, Galileo E5 single-frequency positioning presents a completely different picture (see Figure 11). After one minute of processing, the coordinates’ standard deviations yield several meters. The deviation decreases very quickly. After 15 minutes of observation, the ambiguity terms are fixed to their integer values, enabling one to get results with an RMS of a few centimeters. This is a very efficient method to achieve a faster convergence of the coordinates to a precise solution.
Precise LEO Orbit Determination with Galileo E5
Satellites in an orbit between 250 and 2,000 kilometers above the Earth’s surface, the so-called low Earth orbiters (LEOs), are nowadays often equipped with a GPS receiver for precise orbit determination (POD). GNSS-derived POD is implemented for many LEO Earth observation missions due to the achievable accuracy of a few centimeters and the continuity of measurements, which no other POD technique — for example, satellite laser ranging (SLR) or Doppler Orbitography and Radio-positioning Integrated by Satellite (DORIS) — is able to deliver.
Table 2 summarizes selected LEO mission types, their typical orbital heights, POD techniques currently used, and the mission POD’s typical accuracy requirements. Many of these satellite missions are designed for scientific purposes, while others are operated on a commercial basis, e.g., optical (high-resolution) missions. The decision to use a single- or dual-frequency GNSS receiver on these LEOs is mainly driven by the orbit accuracy requirements, which are dependent on the mission goals, but cost is also a factor: can the mission’s budget support the cost of a dual-frequency receiver? And the price of the receiver is not the only cost factor; depending on the type of GNSS receiver chosen, many other factors have to be adapted accordingly (e.g., power, downlink bandwidth).
The CPC (code-plus-carrier) method as described earlier is suitable for single-frequency LEO POD as well. Especially for LEOs with an excellent pseudorange quality (e.g., GRACE B; see the article by R. Kroes cited in the Additional Resources section), one may reach decimeter accuracy for the orbits. The Galileo E5 signal therefore offers an interesting alternative to GPS receivers. The accuracy can be improved over GPS L1-only receivers, and a Galileo E5 receiver could save costs compared to a dual-frequency GPS receiver while still meeting high-accuracy POD requirements.
Looking over the list in Table 2, the first two mission types — gravity and altimetry — are not suitable for a single-frequency E5 receiver because the accuracy requirements are too high. Neither are radio occultation missions suitable, because a dual-frequency GNSS receiver is essentially needed for the radio occultation technique. Satellite operators of SAR/InSAR and optical missions with panchromatic sensors would, however, be potential users of such a single-frequency E5 receiver. Typically, such missions are operated by space agencies (e.g., ESA, NASA, CNES, DLR) and commercial satellite operators (e.g., SPOT S.A., GeoEye).
POD Test Case
In order to test the potential of E5 for LEO POD, one has to simulate data because all active spaceborne receivers are GPS-only instruments. We selected the ENVISAT mission as a test case, because it represents a typical Earth observation mission. The precise orbits were provided by the European Space Agency (ESA) and used for the generation of synthetic data. Two days of GPS+Galileo data (60-second sampling) have been simulated (December 3, 2003, and February 11, 2004) based on the actual GPS constellation on these days and a Walker constellation for Galileo.
A version of the Bernese GPS Software tailored for LEO POD has been used to generate precise orbits of ENVISAT based on the simulated GPS+Galileo data. The orbits are derived from undifferenced observations, meaning that no additional data from ground tracking stations were needed for 71the processing. Two different orbit types were generated for this test — a reduced-dynamic and a kinematic orbit:
- A kinematic orbit is an ephemeris at discrete measurement epochs (see Figure 12). Kinematic positions are fully independent of the force models used for LEO POD. The analysis technique is, in principle, the same as an epoch-wise point positioning of a moving station on the Earth’s surface.
- In the case of the reduced-dynamic orbits (Figure 12), the satellite trajectory is mainly given through modeling the forces acting on the satellite (a particular solution of the equation of motion). The strength of the force models is reduced, to some extent, by additional empirical parameters.
Both types of orbits were generated because kinematic positioning is very sensitive to data-quality issues so that the improvement in the pseudorange quality for the Galileo E5 frequency can be made visible very clearly. In the case of the reduced-dynamic orbit determination technique, the resulting orbit benefits from the physical force model and the orbits are of the highest quality.
Results
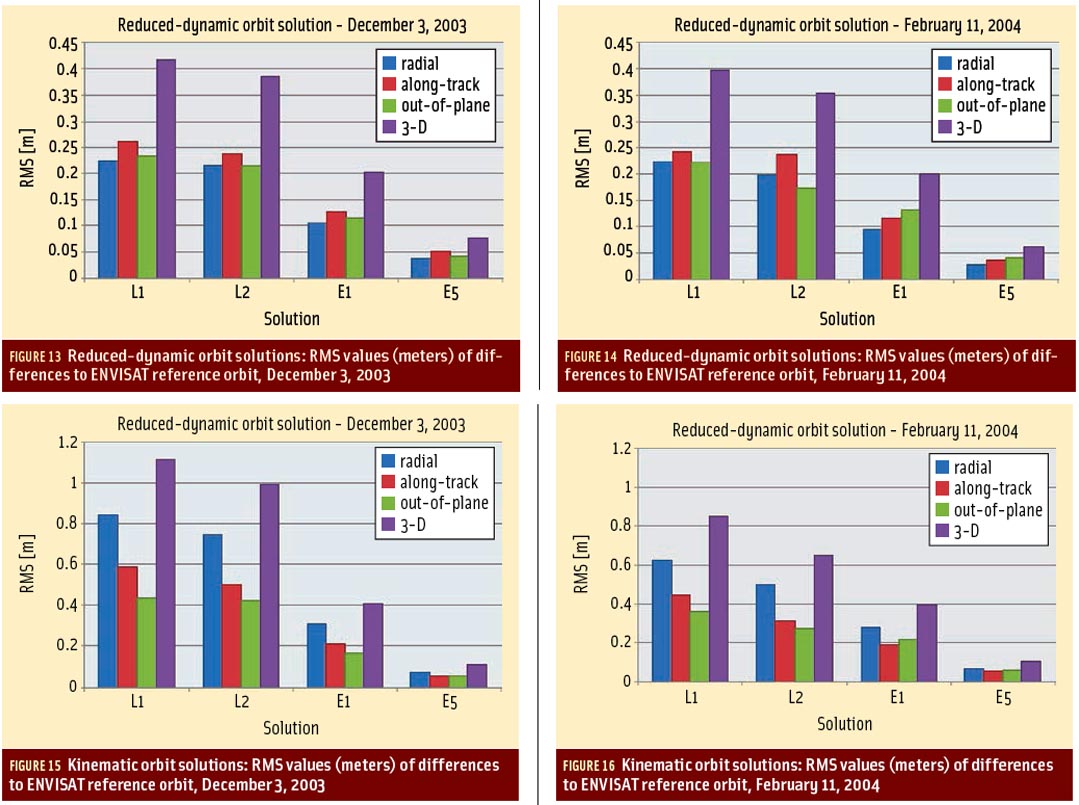
We generated single-frequency CPC solutions for the four main frequencies (GPS L1, L2, and Galileo E1, E5) and compared the resulting orbits to the reference solution from ESA, which had been used to generate the synthetic data. The RMS values of the differences in radial, along-track, and out-of-plane directions as well as in 3-D are shown in Figure 13 and Figure 14 for the reduced-dynamic orbits. Figure 15 and Figure 16 show the corresponding RMS values for the kinematic orbits. The Galileo E5 solution obviously is the best in all cases, with 5–7 centimeters 3-D RMS for the reduced-dynamic and about 10 centimeters 3-D RMS for the kinematic orbits.
Figure 17 shows the orbit differences in the out-of-plane direction between the kinematic orbit derived with L2 and the reference orbit as well as between the kinematic orbit derived with E5 and the reference orbit. Besides the systematic differences that are of no importance here, one may notice the very small noise structure of the differences for the E5 solution compared to the differences for the L2 solution. The reason is the low noise characteristic of E5 and confirms the excellent performance of this observable for a single-frequency approach.
These initial orbit results derived from synthetic data clearly show the potential that the Galileo E5 frequency offers for LEO POD missions. Accuracies of 5 centimeters for reduced-dynamic orbits and 10 centimeters for kinematic orbits are a great success for single-frequency orbit solutions, which have never been achieved with GPS L1. Many future LEO missions may profit in terms of money, downlink bandwidth, and power from such a single-frequency Galileo receiver because the need for a more expensive dual-frequency receiver may be avoided completely.
Conclusion
This article assessed the performances of a single-frequency positioning approach using the potential of the Galileo E5 (AltBOC) broadband signal. Due to its very low code range noise and the even lower multipath influence on the positioning solution (compared to common signals like GPS L1), the combination of Galileo E5 code-plus-carrier measurements is able to achieve accurate positioning results.
The tests that we performed in this research showed that 3D accuracy of a few centimeters (2-3 centimeters) can be achieved with Galileo E5 CPC single-frequency positioning. Compared to the results with GPS L1 or L5 (GPS L1, 10–20 centimeters; L5, 3–6 centimeters), one can see the potential of using Galileo E5. A drawback to the method is the long convergence time (20–30 minutes) to get precise coordinates and achieve an accurate solution. This should be properly addressed in future studies by employing a filtering technique.
Further tests showed that carrier phase processing is still more accurate (by an order of 10) than the CPC single-frequency approach using Galileo E5. However, this kind of processing requires multi-frequency receivers that are more expensive than single-frequency receivers.
Nonetheless, not all precise GNSS applications require precision at millimeter level or in real-time. So, the single-frequency positioning approach with Galileo E5 can fill a niche between carrier phase processing (millimeter level) and the usual single-frequency positioning (decimeter level). In addition, due to the convergence time required to achieve higher accuracy, the E5 approach seems to be suited for monitoring activities in which precise coordinates are only needed to detect changes after a prolonged period of observation.
The CPC method described in this article is also suitable for single-frequency LEO POD. Especially for LEOs with an excellent code pseudo range quality such as GRACE B, one may reach decimeter accuracy for the orbits. This special characteristic of the Galileo E5 signal should make a single-frequency receiver based on it an interesting alternative to the current dual-frequency GPS receivers.
Acknowledgements
The content of the paper was generated within the project “SX5 – Scientific Service Support Based on Galileo E5 Receivers,” which received funding from the European Union within the 7th Framework Programme. It is supervised by the European GNSS Agency (GSA) and coordinated by the Institute of Space Technology and Space Applications, Universität der Bundeswehr München.
Additional Resources
[1] Bock, H., and A. Jaggi, R. Dach, S. Schaer, and G. Beutler, “GPS Single-Frequency Orbit Determination for Low Earth Orbiting Satellites,” Advances in Space Research, Vol. 43, No. 5, pp. 783-791, 2008
[2] Dach, R., and U. Hugentobler, P. Fridez, and and M. Meindl, M. (Eds.), “Bernese GPS Software Version 5.0“, Astronomical Institute, University of Bern, Switzerland, January 2007
[3] Erker, S., and S. Tholert, J. Furthner, and M. Meurer, “The New GPS Signal,” Proceedings of IAIN 2009, Stockholm, Sweden, 2009
[4] Hatch, R., “The Synergism of GPS Code and Carrier Measurements,” Proceedings of the Third International Geodetic Symposium on Satellite Doppler Positioning, Las Cruces, New Mexico, Vol. II, pp. 1213-1232, February 8–12, 1982
[5] Irsigler, M., Multipath Propagation, Mitigation and Monitoring in the Light of Galileo and the Modernized GPS, Dissertation, University FAF Munich, 2008
[6] Jaggi, A., and U. Hugentobler, and G. Beutler, “Pseudo-Stochastic Orbit Modeling Techniques for Low-Earth Orbiters,” Journal of Geodesy, Vol. 80, No. 1, pp. 47–60, 2006
[7] Kroes, R., “Precise Relative Positioning of Formation Flying Spacecraft using GPS,” Optima Grafische Communicatie, Rotterdam, 2006
[8] Le, A. Q. and C. Tiberius, “Single-Frequency Precise Point Positioning with Optimal Filtering,” GPS Solutions, Vol. 11, pp. 61–69, 2007
[9] Lambiel, C., and R. Delaloye, “Contribution of Real-time Kinematic GPS in the Study of Creeping Mountain Permafrost: Examples from the Western Swiss Alps,” Permafrost and Periglacial Processes, 15: 229-241, 2004, published online in Wiley InterScience. DOI: 10.1002/ppp.496
[10] Misra, P. and P. Enge, Global Positioning System: Signals, Measurements, and Performance, Ganga-Jamuna Press, 1st edition, p. 390, 2001
[11] Schüler, T., and T. H. Diessongo, and Y. Poku-Gyamfi, Y., “Precise Ionosphere-Free Single-Frequency GNSS Positioning”, GPS Solutions, Vol. 2–2011, pp. 139–147, 2010
[12] Simsky, A., and D. Mertens, J. M. Sleewaegen, W. De Wilde, S. S. Navigation, and M. Hollreiser, M. “Multipath and Tracking Performance of Galileo Ranging Signals Transmitted by GIOVE-B,” Proceedings of the 21st International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GNSS 2008), Vol. 3, pp. 1525–1536, 2008
[13] Švehla, D. and M. Rothacher, “Kinematic Precise Orbit Determination for Gravity Field Determination,” Sansò F. (Ed.), A Window on the Future of Geodesy, Vol. 128, pp. 181–188, Springer, Berlin, 2005
[14] Teunissen, P. J. G., and P. J. DeJonge, and C. C. J. M. Tiberius, “A New Way to Fix Carrier Phase Ambiguities,” GPS World, pp. 58–61, April 1995
[15] Yunck, T. P., “Orbit Determination,” in Parkinson, B. W., and J. J. Spilker, (Eds.), Global Positioning System—Theory and Applications, AIAA, Washington D.C., 1996